|
|
| Name: | Sapphire Crystal Lens (Ball/Half-ball) |
| P/N: | SPL-HB-CO-6.5-U | | Keywords: | Sapphire,Half-ball Lens,6.5mm,C-normal Orientation |
| Unit Price: |
S$420.00/pc
|
| Available: |
Call us
|
| Request: |
Add to Request List |
| Introduction: | As the hardest one of all the oxide crystals, Sapphire has the combination of optical and physical properties that make it the best choice for a variety of demanding applications. Sapphire maintains its strength even at high temperature. It has good thermal properties, excellent electrical and dielectric properties and is resistant to chemical attack. These properties encourage the use of Sapphire in aggressive environments where long-term reliability, high optical transmission or good strength is required.
Ball lenses are highly polished spheres made of optically transparent homogeneous materials. Carefully controlled manufacturing process produces spheres with precise diameters and unsurpassed surface quality. Ball lenses are great tools for improving signal coupling between fibers, emitters and detectors. |
|
|
| Substrate Material |
Sapphire Crystal |
| Outer Diameter |
6.5mm, -0.1/0mm |
| Centre Thickness |
3.25mm, +/-0.05mm |
| Lens Design |
Half-ball ; Hemi-spherical surface |
| Radius of Curvature |
3.25mm ,-0.1/0mm |
| Edge Surface |
None; Edge thickness (ET) = 0, specially processed |
| Surface Quality |
Spherical surface:
40/20 scratch and dig; Surface accuracy: <lambda/4 @ 633nm; Clear aperture: >90%
Plano Surface:
20-10S/D; <lambda/4 @633nm over surface; Clear aperture: >90% |
| Coating |
Uncoated on both surfaces |
|
Chamfer/Chip: |
0.2mmx45deg protection chamfer; Chip <0.1mm |
| Orientation |
C-normal at Plano surface only |
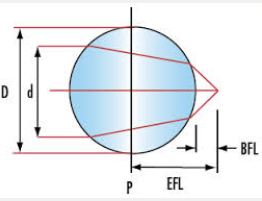
The effective focal length of a ball lens is very simple to calculate since there are only two variables: the ball lens diameter, D, and the index of refraction, n. The effective focal length is measured from the center of the lens. Therefore, the back focal length can also be easily calculated.
BFL = F - [D/2] EFL = nD/[4(n - 1)]
The Numerical Aperture, NA, of a ball lens is dependent on the focal length of the ball and on the input diameter, d. Since spherical aberration is inherent in ball lenses the following equation begins to fall off as d/D increases.
NA = [2d(n - 1)]/nD
When coupling light from a laser into a fiber, the choice of the ball is dependent on the NA of the fiber and the diameter of the laser beam. The diameter of the laser beam is used to determine the NA of the ball lens. The NA of the ball lens must be less than or equal to the NA of the fiber in order to couple all of the light into the fiber. The ball lens is placed directly onto the fiber.
To couple light from one fiber to another fiber of similar NA, two identical ball lenses are used. Place the two lenses in contact with the fibers.
|
Optical Substrates
Optical Lenses
Spherical Plano Convex Lenses
